Graph, Wall, Tome
Background
Imagine: It's 1915, and you've made one of the greatest discoveries in hundreds of years. You visit your mother and show her your work:
$$R_{\mu v}-\frac{1}{2}Rg_{\mu v} = 8 \pi T_{\mu v}$$
'That's nice dear', she responds, unaware of the implications of your discovery.
The problem, is that although this equation carries with it the secrets of gravity, to a layman it is merely a bunch of letters.
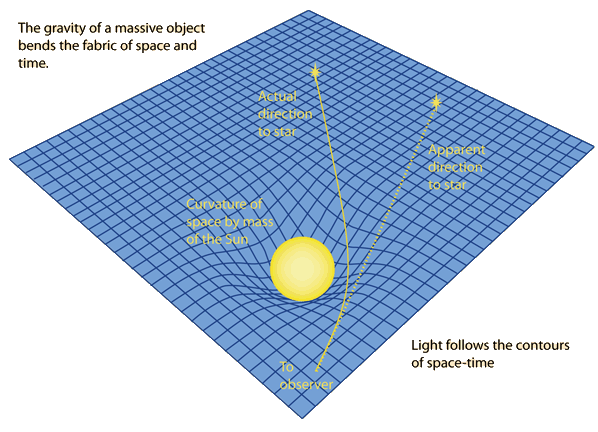
Now, consider this single image.
Instantly, the meaning becomes clear. Gravity warps space(time), and matter, planets, and even light follows the curved path.
Fundamental physics is an unknown world to most people. Equations, symbols, and incomprehensible terms abound, and unless you've studied post-grad mathematics and physics, this world is inaccessible to you.
Although there are several great resources to map the way toward complete understanding. Most people will not undertake the journey to understand the source code to the world that we all inhabit.
The Assignment
There are 3 different pieces of the puzzle that Eric Weinstein pointed to during a call on Discord: graph, wall and tome. Please hold on to this information, that is they’re not public yet, until they come up in a dedicated future episode on The Portal. In the meantime, feel free to try to figure out how they fit together and what you can do with them in order to understand reality. Also, trying to answer the questions at the end helps you get started.
This was first collected in a Google Doc titled Graph, Wall, Tome - Problem Solving.
The Graph
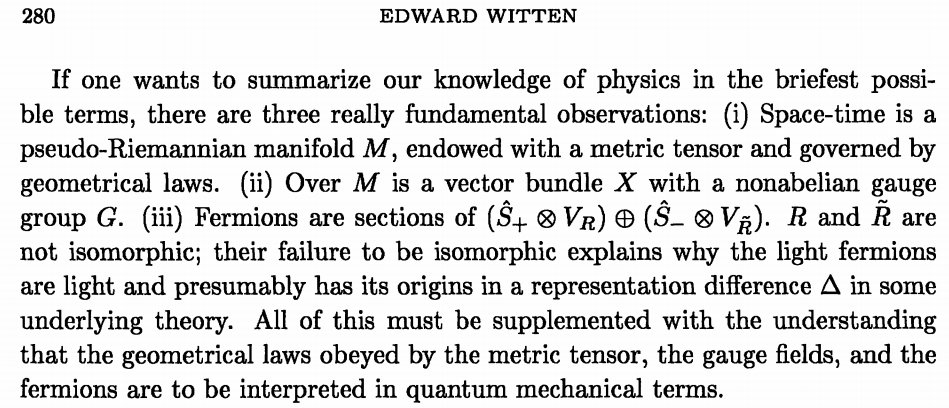
The paragraph by Edward Witten as posted by Eric via Twitter.
However, there are 3 flaws with it:
- In (ii), “vector bundle X” should be changed to principal G-bundle.
- Also in (ii), “nonabelian gauge group G” should be changed to nonabelian structure group G.
- In (iii), [math]\displaystyle{ \ R }[/math] and [math]\displaystyle{ \tilde R }[/math] should be (complex) linear representations of G and so they are not equivalent.
Expansion
Converted to text and re-written according to 'flaws'
If one wants to summarise our knowledge of physics in the briefest possible terms, there are three really fundamental observations:
- Spacetime is a pseudo-Riemannian manifold M, endowed with a metric tensor and governed by geometrical laws.
- Over M is a principal bundle $$P_{G}$$ with a non-abelian structure group G.
- Fermions are sections of $$(\hat{S}_{+} \otimes V_{R}) \oplus (\hat{S}\_ \otimes V_{\bar{R}})$$. $$R$$ and $$\bar{R}$$ are not isomorphic; their failure to be isomorphic explains why the light fermions are light.
- Add something about Higgs
All of this must be supplemented with the understanding that the geometrical laws obeyed by the metric tensor, the gauge fields, and the fermions are to be interpreted in quantum mechanical terms.
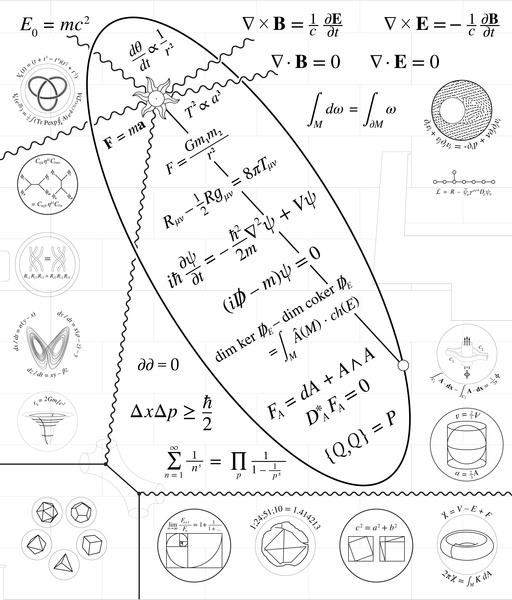
The Wall
Eric talked about some of the important equations on the wall. There are 2 different recorded versions of the conversation if you want to listen to it.
Expansion
1: Einstein's General Relativity equation:
- $$R_{\mu v}-\frac{1}{2}Rg_{\mu v} = 8 \pi T_{\mu v}$$
2: Maxwell's equations:
- $$\nabla \times \mathbf{B} = \frac{1}{c} \frac{\partial \mathbf{E}}{\partial t}$$
- $$\nabla \times \mathbf{E} = -\frac{1}{c} \frac{\partial \mathbf{B}}{\partial t}$$
- $$\nabla \cdot \mathbf{B} = 0$$
- $$\nabla \cdot \mathbf{E} = 0$$
2: Yang-Mills equations:
- $$d^*_A F_A \propto J$$
3: Dirac equation:
- $$(i \not{D}_A - m)\psi = 0$$
4: Klein-Gordon equation:
- $$\frac{1}{c^2} \frac{\partial^2}{\partial t^2} \psi - \nabla^2 \psi + \frac{m^2 c^2}{\hbar^2} \psi = 0$$
Einstein's mass-energy equation:
- $$E = mc^2$$
Kepler's 2nd law:
- $$\frac{d\theta}{dt} \propto \frac{1}{r^2}$$
Newton's force-acceleration equation:
- $$\mathbf{F} = m\mathbf{a}$$
Keplers 3rd law:
- $$T^2 \propto a^3$$
Newtons gravitational law:
- $$F = \frac{G m_1 m_2}{r^2}$$
Schrodinger's equation:
- $$i \hbar \frac{\partial \psi}{\partial t} = - \frac{\hbar^2)}{2 m} \nabla^2 \psi + V \psi$$
Atiyah-Singer theorem:
- $$dim\, ker \not{D}_E - dim \, coker \not{D}_E = \int_M \hat{A}(M) \cdot ch(E)$$
Defining relation of supersymmetry:
- $$\{Q,Q\} = P$$
Stokes' theorem:
- $$\int_M d\omega = \int_{\partial M}\omega$$
The boundary of a boundary is zero:
- $$\partial\partial = 0$$
Heisenberg's indeterminacy relation:
- $$\Delta x \Delta p \geq \frac{\hbar}{2}$$
Euler's formula for Zeta-function:
- $$\sum\limits_{n=1}^{\infty} \frac{1}{n^{s}} = \prod\limits_{p} \frac{1}{1 - \frac{1}{p^s}}$$
The Tome
ISBN: 978-0679776314
- Road to Reality by Roger Penrose (2004)
- There appears to be a Kindle Edition that isn't available in the US. If anyone in the community has a way to get a Kindle version of the book, please add it here.
- Purchase the book somehow, then get the pdf here
Questions
Some questions Eric posed related to the assignment:
What is $$F_A$$ geometrically?
What are $$R_{\mu v}$$ and $$R$$ geometrically?
Einstein field equaitions Einstein’s original publication, Die Feldgleichungen der Gravitation, in English
$$R$$
$$R$$ is a scalar value, describing the "curvature of the spacetime manifold" at each point along the manifold. It's based on a concept of 'parallel transport', where you move a vector around some path on the manifold.
$$R$$ can be computed at each point on the manifold, and describes the difference in the vector's angle after following an infinitesimally small path around the neighborhood of that point, vs. what it was originally. The video does a great job of visualizing when and why that vector angle change would happen, with flat vs. curved manifolds.
In the video, they focus first on the curvature of space. Hopefully they incorporate back in curvature in time, because that's less obvious.
$$R_{\mu v}$$
The same video then proceeds to explain $$R_{\mu v}$$. It progresses through some concepts.
Computing length in non-orthogonal bases
First, just describing the length of a vector on a curved space is hard. It is given by:
$$Length^{squared} = g_{11}dX^{1}dX^{1} + g_{12}dX^{1}dX^{2} + g_{21}dX^{2}dX^{1} + g_{22}dX^{2}dX^{2}$$
Some notes:
- This is not Pythagorean theorem, because $$dX^{1}$$ and $$dX^{2}$$ are not perpendicular.
- Instead, looks like a formula to get the diagonal from two opposite vertices in a parallelogram.
- If $$dX^{1}$$ and $$dX^{2}$$ are perpendicular, then $$g_{12}$$ and $$g_{21}$$ would be 0, and we would get $$Length^{squared} = g_{11}(dX^{1})^{2} + g_{22}(dX^{2})^{2}$$
- See: the video @ 14m27s
Computing vector rotation due to parallel transport
Then, they show parallel transport when following a parallelogram, but over a curved 3D manifold. To compute the vector rotation by components, they show:
$$dV^{1} = dX^{1}dX^{2} (V^{1}R^{1}_{112} + V^{2}R^{1}_{212} + V^{3}R^{1}_{312})$$
$$dV^{2} = dX^{1}dX^{2} (V^{1}R^{2}_{112} + V^{2}R^{2}_{212} + V^{3}R^{2}_{312})$$
$$dV^{3} = dX^{1}dX^{2} (V^{1}R^{3}_{112} + V^{2}R^{3}_{212} + V^{3}R^{3}_{312})$$
or, using $$i$$ to summarize across all 3 components (difference vectors):
$$dV^{i} = dX^{1}dX^{2} (V^{1}R^{i}_{112} + V^{2}R^{i}_{212} + V^{3}R^{i}_{312})$$
or , using $$j$$ to index over all 3 components (original vector):
$$dV^{i} = dX^{1}dX^{2} \Sigma_{j} [(V^{j}R^{i}_{j12}]$$
See: the video @ 19m33s
Open questions:
- Why a parallelogram?
- How to properly overlay the parallelogram onto the 3d manifold, in order to do the parallel transport?
- How does this relate to the length computation above?
Putting it all together
Now, moving to 4D, we can compute $$R_{\mu v}$$ as:
$$R_{00} = R^{0}_{000} + R^{1}_{010} + R^{2}_{020} + R^{3}_{030}$$
$$R_{10} = R^{0}_{100} + R^{1}_{110} + R^{2}_{120} + R^{3}_{130}$$
$$R_{01} = R^{0}_{001} + R^{1}_{011} + R^{2}_{021} + R^{3}_{030}$$
etc.
Indexing i over all 4 component vectors / dimensions, we get:
$$R_{00} = \Sigma_{i} R^{i}_{0i0}$$
$$R_{10} = \Sigma_{i} R^{i}_{1i0}$$
$$R_{01} = \Sigma_{i} R^{i}_{0i1}$$
etc.
Summarizing on $$\mu$$, we get:
$$R_{\mu 0} = \Sigma_{i} R^{i}_{\mu i0}$$
$$R_{\mu 1} = \Sigma_{i} R^{i}_{\mu i1}$$
etc
Summarizing on $$v$$, we get:
$$R_{\mu v} = \Sigma_{i} R^{i}_{\mu iv}$$
Open questions:
- If we hadn't moved from 3D to 4D, what would this all have looked like?
- What does this have to do with the parallelogram?
- Why are there two indices?
How do they relate?
What does this have to do with Penrose Stairs?
We’ve heard Eric talk about Penrose stairs and spinors - essentially phenomena where you cannot return to the original state through a 360 degree rotation, but require a 720 degree rotation.
What are “Horizontal Subspaces” and what do they have to do with Vector Potentials or Gauge fields?
From theplebistocrat:
Generally, we're wanting to understand how fermions arise from - or are embedded within / upon - topological "spaces" that have distinct rules which govern operations within those topological spaces, and then how those rules produce higher dimensional operations in corresponding spaces.
Just intuitively, and geometrically speaking, the image that I'm getting when describing all of this and trying to hold it in my head is the image of a sort of Penrose Tower of Babel, where the fundamental underlying structures reach upwards (but also downwards and inwards?) before reaching a critical rotation that corresponds to a collapse of structure into a higher dimensional fiber bundle.
But doesn't this require the symmetry break? How is left and right rotation in a subspace transformed into verticality? This is a crazy rabbit hole, friends. Keep your chins up. Let me know if this was helpful or leading astray.