Jones polynomial: Difference between revisions
No edit summary |
No edit summary |
||
| Line 4: | Line 4: | ||
From Wikipedia, the free encyclopedia | From Wikipedia, the free encyclopedia | ||
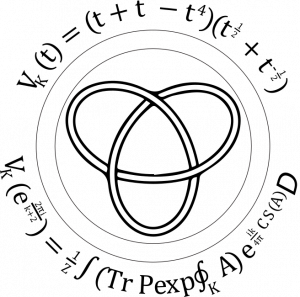
In the mathematical field of knot theory, the Jones polynomial is a knot polynomial discovered by Vaughan Jones in 1984. Specifically, it is an invariant of an oriented knot or link which assigns to each oriented knot or link a Laurent polynomial in the variable | In the mathematical field of knot theory, the Jones polynomial is a knot polynomial discovered by Vaughan Jones in 1984. Specifically, it is an invariant of an oriented knot or link which assigns to each oriented knot or link a Laurent polynomial in the variable $$ t^{1/2} $$ with integer coefficients. | ||
==Resources:== | ==Resources:== | ||
Revision as of 18:09, 4 February 2020
From Wikipedia, the free encyclopedia
In the mathematical field of knot theory, the Jones polynomial is a knot polynomial discovered by Vaughan Jones in 1984. Specifically, it is an invariant of an oriented knot or link which assigns to each oriented knot or link a Laurent polynomial in the variable $$ t^{1/2} $$ with integer coefficients.