Graph, Wall, Tome: Difference between revisions
m (→The Graph) |
m (→The Graph) |
||
| Line 64: | Line 64: | ||
<blockquote> | <blockquote> | ||
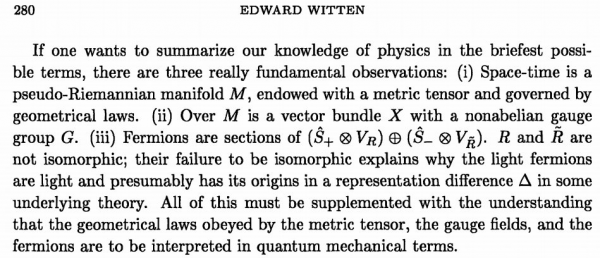
If one wants to summarise our knowledge of physics in the briefest possible terms, there are three really fundamental observations: | If one wants to summarise our knowledge of physics in the briefest possible terms, there are three really fundamental observations: | ||
(i) Spacetime is a pseudo-Riemannian manifold | (i) Spacetime is a pseudo-Riemannian manifold $$M$$, endowed with a metric tensor and governed by geometrical laws. | ||
(ii) Over M is a vector bundle $$X$$ with a nonabelian gauge group : $$G$$. | (ii) Over M is a vector bundle $$X$$ with a nonabelian gauge group : $$G$$. | ||
(iii) Fermions are sections of $$(\hat{S}{+} \otimes V{R}) \oplus (\hat{S}_ \otimes V_{\bar{R}})$$. $$R$$ and $$\bar{R}$$ are not isomorphic; their failure to be isomorphic explains why the light fermions are light and presumably has its origins in representation difference $$\Delta$$ in some underlying theory. | (iii) Fermions are sections of $$(\hat{S}{+} \otimes V{R}) \oplus (\hat{S}_ \otimes V_{\bar{R}})$$. $$R$$ and $$\bar{R}$$ are not isomorphic; their failure to be isomorphic explains why the light fermions are light and presumably has its origins in representation difference $$\Delta$$ in some underlying theory. | ||
| Line 84: | Line 84: | ||
If one wants to summarise our knowledge of physics in the briefest possible terms, there are three really fundamental observations: | If one wants to summarise our knowledge of physics in the briefest possible terms, there are three really fundamental observations: | ||
# [https://en.wikipedia.org/wiki/Spacetime Spacetime] is a [https://en.wikipedia.org/wiki/Pseudo-Riemannian_manifold pseudo-Riemannian manifold] | # [https://en.wikipedia.org/wiki/Spacetime Spacetime] is a [https://en.wikipedia.org/wiki/Pseudo-Riemannian_manifold pseudo-Riemannian manifold] $$M$$, endowed with a [[metric tensor]] and governed by [https://en.wikipedia.org/wiki/Geometry geometrical laws]. | ||
# Over $$M$$ is a [https://en.wikipedia.org/wiki/Principal_bundle principal bundle] : $$P_{G}$$, with a [https://en.wikipedia.org/wiki/Non-abelian_group non-abelian structure group] : $$G$$. | # Over $$M$$ is a [https://en.wikipedia.org/wiki/Principal_bundle principal bundle] : $$P_{G}$$, with a [https://en.wikipedia.org/wiki/Non-abelian_group non-abelian structure group] : $$G$$. | ||
# [https://en.wikipedia.org/wiki/Fermion Fermions] are sections of $$(\hat{S}_{+} \otimes V_{R}) \oplus (\hat{S}\_ \otimes V_{\bar{R}})$$. $$R$$ and $$\bar{R}$$ are not [https://en.wikipedia.org/wiki/Isomorphism isomorphic]; their failure to be isomorphic explains why the light fermions are light. | # [https://en.wikipedia.org/wiki/Fermion Fermions] are sections of $$(\hat{S}_{+} \otimes V_{R}) \oplus (\hat{S}\_ \otimes V_{\bar{R}})$$. $$R$$ and $$\bar{R}$$ are not [https://en.wikipedia.org/wiki/Isomorphism isomorphic]; their failure to be isomorphic explains why the light fermions are light. | ||
Revision as of 20:55, 16 April 2020
A Request From Eric
A request: Try to draw the lines through the three. View it as a unified idea:
The paragraph gets edited.
The wall gets defaced and grafittied.
The Sacred Tome gets Re-Written
But follow the skeins through each.
The graph points to the wall.
And the wall to the Tome.
And the Tome leads to the Search.
Background
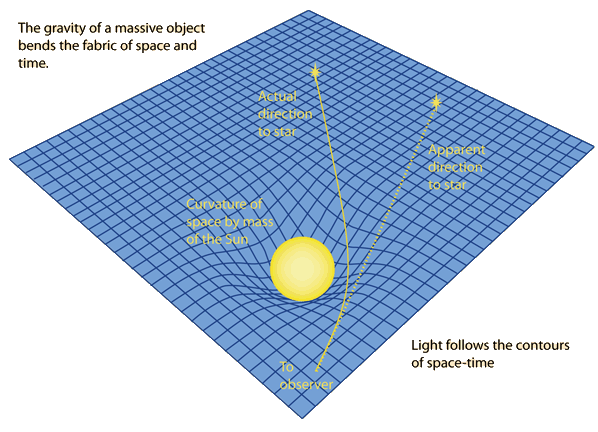
Imagine: It's 1915, and you've made one of the greatest discoveries in hundreds of years. You visit your mother and show her your work:
$$R_{\mu v}-\frac{1}{2}Rg_{\mu v} = 8 \pi T_{\mu v}$$
'That's nice dear', she responds, unaware of the implications of your discovery.
The problem, is that although this equation carries with it the secrets of gravity, to a layman it is merely a bunch of letters and symbols.
Now, consider this single image.
Instantly, the meaning becomes clear. Gravity warps space(time), and matter, planets, and even light follows a path that is curved by the warped geometry.
Fundamental physics is an unknown world to most people. Equations, symbols, and incomprehensible terms abound, and unless you've studied post-grad mathematics and physics, this world is inaccessible to you.
Although there are several great resources to map the way toward complete understanding. Most people will not undertake the journey to understand the source code to the world that we all inhabit.
The GWT Project
Bringing an understanding of fundamental physics is one aim of The Portal.
There currently exist 3 resources that themselves contain all that you need for an almost complete understanding of the world.
- The Graph - A paragraph written by Edward Witten
- The Wall - The iconic wall of Stony Brook University
- The Tome - The book 'The Road to Reality' by Roger Penrose
These resources are available to everyone, but will be sought by almost none. The aim of the GWT project to convert these resources into a medium that can be widely disseminated, and which can not be ignored.
This project will require bi-directional information transfer, and the minds of people with many different aptitudes.
- We need mathematicians, topologists, geometers, and physicists to understand these resources, and all of their implications.
- We need explainers and educators, to convey this information to a wider audience, and
- We need artists, linguists, and programmers to create intuitive visualisations.
The Portal will create a community of people, working together to achieve these aims.
Success will generate yet further insights, opening up a more fundamental understanding of the nature of reality - for the individuals involved, and - for humanity as a whole.
This was first collected in a Google Doc titled Graph, Wall, Tome - Problem Solving.
The Graph
If one wants to summarise our knowledge of physics in the briefest possible terms, there are three really fundamental observations: (i) Spacetime is a pseudo-Riemannian manifold $$M$$, endowed with a metric tensor and governed by geometrical laws. (ii) Over M is a vector bundle $$X$$ with a nonabelian gauge group : $$G$$. (iii) Fermions are sections of $$(\hat{S}{+} \otimes V{R}) \oplus (\hat{S}_ \otimes V_{\bar{R}})$$. $$R$$ and $$\bar{R}$$ are not isomorphic; their failure to be isomorphic explains why the light fermions are light and presumably has its origins in representation difference $$\Delta$$ in some underlying theory. All of this must be supplemented with the understanding that the geometrical laws obeyed by the metric tensor, the gauge fields, and the fermions are to be interpreted in quantum mechanical terms.
The original publication Physics and Geometry by Edward Witten can be accessed via the CERN Document Server. The paragraph is found at the bottom of page 20.
Eric Weinstein suggested several alterations, that have been included below:
- In (ii), “vector bundle X” should be changed to principal G-bundle.
- Also in (ii), “nonabelian gauge group G” should be changed to nonabelian structure group G.
- In (iii), [math]\displaystyle{ \ R }[/math] and [math]\displaystyle{ \tilde R }[/math] should be (complex) linear representations of G and so they are not equivalent.
- He mentioned that some info was not required, and that higgs is remarkably absent.
This is a modified version of the paragraph by Edward Witten as posted by Eric via Twitter.
If one wants to summarise our knowledge of physics in the briefest possible terms, there are three really fundamental observations:
- Spacetime is a pseudo-Riemannian manifold $$M$$, endowed with a metric tensor and governed by geometrical laws.
- Over $$M$$ is a principal bundle : $$P_{G}$$, with a non-abelian structure group : $$G$$.
- Fermions are sections of $$(\hat{S}_{+} \otimes V_{R}) \oplus (\hat{S}\_ \otimes V_{\bar{R}})$$. $$R$$ and $$\bar{R}$$ are not isomorphic; their failure to be isomorphic explains why the light fermions are light.
- Add something about Higgs
All of this must be supplemented with the understanding that the geometrical laws obeyed by the metric tensor, the gauge fields, and the fermions are to be interpreted in quantum mechanical terms.
The Wall
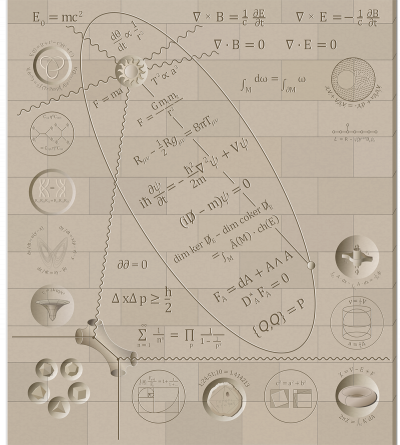
This image is carved into a wall at Stony Brook University. It contains many of the most fundamental equations of physics, providing a formulaic representation of all reality.
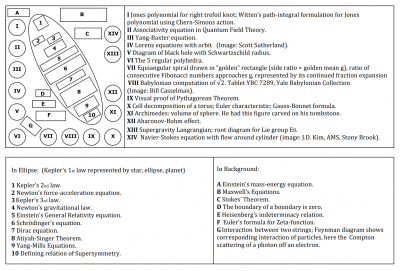
Several of the equations have been identified as having direct connections to statements in 'The Graph' (identified by numbers)
Key and Explanations
The following should be completed according to the list of explanations on this page: http://scgp.stonybrook.edu/archives/6264
- I. Jones polynomial for right trefoil knot; Witten’s path-integral formulation for Jones polynomial using Chern-Simons action
- II. Feynmann Diagram illustrating Associativity equation in Quantum Field Theory
- III. Yang-Baxter equation
- IV. Lorenz Attractor: Lorenz equations with orbit
- V. Diagram of a black hole with Schwarzschild radius
- VI. The five regular polyhedra
- VII. Equiangular spiral drawn in "golden" rectangle (side ratio = golden mean g), ratio of consecutive Fibonacci numbers approaches g, represented by its continued fraction expansion.
- VIII.Babylonian computation of the square root of 2
- IX. Visual proof of the Pythagorean Theorem
- X. Cell decomposition of torus; Euler characteristic; Gauss-Bonnet formula.
- XI. Archimedes: On the Sphere and Cylinder.
- XII. Aharanov-Bohm effect
- XIII.Supergravity Langangian; root diagramm for Lie group E8
- XIV. Navier-Stokes equation with flow around cylinder.
- 0. In Ellipse: (Kepler's 1st law represented by star, ellipse, planet)
- 1. Kepler's 2nd law
- 2. Newton's force-acceleration equation
- 3. Kepler's 3rd law
- 4. Newton's gravitational law
- 5. Einstein's General Relativity equation
- 6. Schrödinger's equation
- 7. Dirac equation
- 8. Atiyah-Singer Theorem
- 9. Yang-Mills equations
- 10. Defining relation of Supersymmetry
- A. Einstein’s mass-energy equation
- B. Maxwell's Equations
- C. Stoke's Theorem
- D. The boundary of a boundary is zero
- E. Heisenberg's indeterminacy relation
- F. Euler's formula for Zeta-function
- G. Interaction between two string; Feynman diagram shows corresponding interaction of particles, here the Compton scattering of a photon off an electron.
Defacing the Wall
What should be in and what should be out of the future wall? Let's determine that[here].
Eric talked about some of the important equations on the wall. There are 2 different recorded versions of the conversation if you want to listen to it.
The Tome
This book by Roger Penrose contains a comprehensive account of the physical universe.
To gain an understanding and intuition for the information contained in 'The Graph', and 'The Wall', reading this book will provide a great head-start.
With 34 chapters spread over 1000 pages, including diagrams, equations, and descriptions, there are multiple avenues for understanding all concepts.
See our study notes
Book Details
- ISBN: 978-0679776314
- Road to Reality by Roger Penrose (2004)
- There appears to be a Kindle Edition that isn't available in the US. If anyone in the community has a way to get a Kindle version of the book, please add it here.
- Purchase the book somehow, then get the pdf here
Methodology
- Create/assemble resources that allow uninitiated to quickly and easily get up to speed with state of project.
- Create/assemble resources that provide analogies for more complex principles - to demonstrate a key feature of the principle.
- Create/assemble resources that provide a geometric intuition for the equations of fundamental physics - Geometric interpretations are most amenable to being visually represented.
- Create/assemble resources that allow the geometric visualisation/intuition for principles of foundational physics.
- Rinse and repeat.
Project Files
A working area for the project is located in a google drive.
It includes a list of tasks that can be worked on by individuals or groups of individuals. (add a comment to let people know if you're working on something)
This file also has an area to list specific areas of aptitude or interest for those people who are not sure how to contribute.
Accelerators
Accelerators: 2 minute guides to fundamental principles.