Jones polynomial: Difference between revisions
(Created page with "From Wikipedia, the free encyclopedia In the mathematical field of knot theory, the Jones polynomial is a knot polynomial discovered by Vaughan Jones in 1984. Specifically, i...") |
No edit summary |
||
| (7 intermediate revisions by 4 users not shown) | |||
| Line 1: | Line 1: | ||
<div class="floatright" style="text-align: center"> | |||
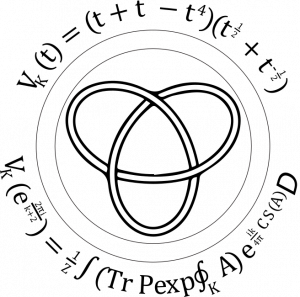
[[File:Jones_polynomial.png|center|class=shadow|300px]] | |||
</div> | |||
'''Vaughan Jones''' (b. 1952) | |||
'''''Jones polynomial''''' 1984 | |||
Resources: | In the mathematical field of [https://en.wikipedia.org/wiki/Knot_theory knot theory], the Jones polynomial is a [https://en.wikipedia.org/wiki/Knot_polynomial knot polynomial] discovered by [https://en.wikipedia.org/wiki/Vaughan_Jones Vaughan Jones] in 1984. Specifically, it is an invariant of an oriented knot or link which assigns to each oriented knot or link a [https://en.wikipedia.org/wiki/Laurent_polynomial Laurent polynomial] in the variable <math>t^{1/2}</math> with integer coefficients. | ||
==Resources:== | |||
*[https://en.wikipedia.org/wiki/Jones_polynomial Jones polynomial] | *[https://en.wikipedia.org/wiki/Jones_polynomial Jones polynomial] | ||
*[https://en.wikipedia.org/wiki/Jones_polynomial#Link_with_Chern%E2%80%93Simons_theory Chern Simons theory] | *[https://en.wikipedia.org/wiki/Jones_polynomial#Link_with_Chern%E2%80%93Simons_theory Chern Simons theory] | ||
Discussion: | ==Discussion:== | ||
[[Category:Pages for Merging]] | |||
Latest revision as of 16:45, 19 February 2023
Vaughan Jones (b. 1952)
Jones polynomial 1984
In the mathematical field of knot theory, the Jones polynomial is a knot polynomial discovered by Vaughan Jones in 1984. Specifically, it is an invariant of an oriented knot or link which assigns to each oriented knot or link a Laurent polynomial in the variable [math]\displaystyle{ t^{1/2} }[/math] with integer coefficients.