|
|
| (3 intermediate revisions by 2 users not shown) |
| Line 1: |
Line 1: |
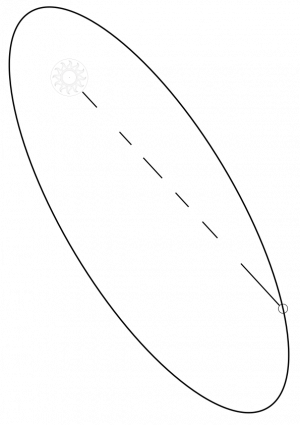
| The orbit of every planet is an ellipse with the Sun at one of the two foci.
| | <div class="floatright" style="text-align: center"> |
| | [[File:Kepler1stlaw.png|center|class=shadow|300px]] |
| | </div> |
| | '''Johannes Kepler''' (b. 1571) |
|
| |
|
| | ''''' Kepler's laws of planetary motion''''' 1609-1619 |
|
| |
|
| Mathematically, an ellipse can be represented by the formula:
| | The orbit of every planet is an ellipse with the Sun at one of the two foci. |
| | |
| $${e r={\frac {p}{1+\varepsilon \,\cos \theta }},}{\displaystyle r={\frac {p}{1+\varepsilon \,\cos \theta }},}$$
| |
| | |
| where $$p$$ is the semi-latus rectum, ε is the eccentricity of the ellipse, r is the distance from the Sun to the planet, and θ is the angle to the planet's current position from its closest approach, as seen from the Sun. So (r, θ) are polar coordinates.
| |
|
| |
|
| For an ellipse 0 < ε < 1 ; in the limiting case ε = 0, the orbit is a circle with the Sun at the centre (i.e. where there is zero eccentricity).
| |
|
| |
|
|
| |
|
| Line 14: |
Line 13: |
| *[https://en.wikipedia.org/wiki/Kepler%27s_laws_of_planetary_motion#First_law_of_Kepler Kepler's 1st law] | | *[https://en.wikipedia.org/wiki/Kepler%27s_laws_of_planetary_motion#First_law_of_Kepler Kepler's 1st law] |
| == Discussion: == | | == Discussion: == |
| | [[Category:Pages for Merging]] |
Latest revision as of 17:38, 1 November 2020
Johannes Kepler (b. 1571)
Kepler's laws of planetary motion 1609-1619
The orbit of every planet is an ellipse with the Sun at one of the two foci.
Resources:[edit]
Discussion:[edit]