Annotating the Wall: Difference between revisions

From The Portal Wiki
No edit summary
No edit summary
 
(10 intermediate revisions by 3 users not shown)
Line 1: Line 1:
<gallery mode="packed" heights=400px>
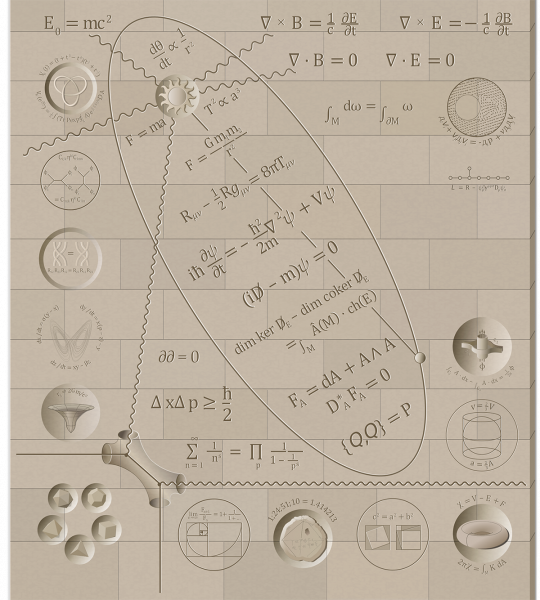
File:Newwall.png|center|class=shadow|400px|The Wall
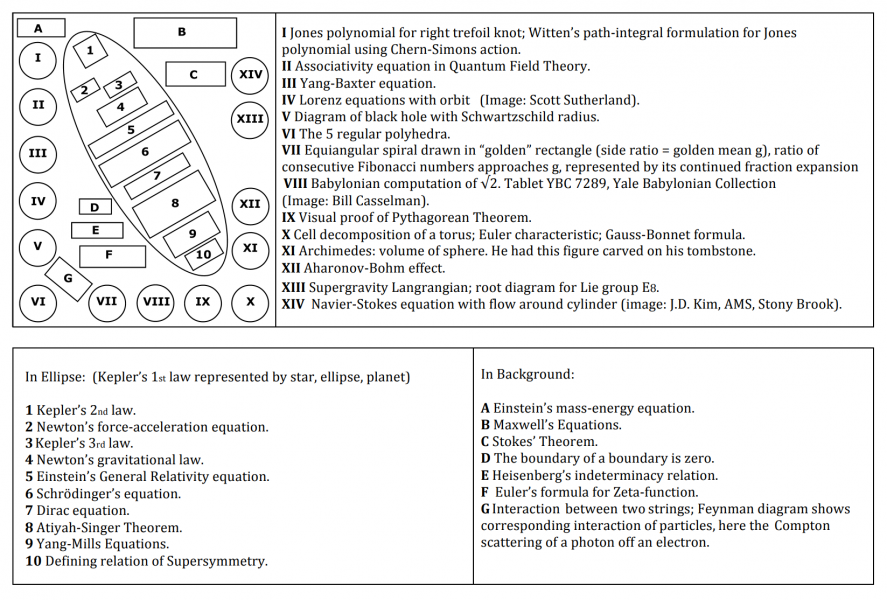
File:Key-to-wall.png|center|class=shadow|400px|Explanations for the Wall
</gallery>


* A [https://dev.theportal.dev/wall/ High Resolution Interactive Version of the Wall] has been created


The following list contains the names of all equations, formulas, and illustrations that are shown on the Wall. The goal is to create a helpful explanation for each element of the list.
The following list contains the names of all equations, formulas, and illustrations that are shown on the Wall. The goal is to create a helpful explanation for each element of the list.


*I.  [[Jones polynomial]] for right trefoil knot; [https://theportal.wiki/wiki/Jones_polynomial Witten’s path-integral formulation] for Jones polynomial using Chern-Simons action
*I.  [[Jones polynomial]] for right trefoil knot; [https://theportal.wiki/wiki/Jones_polynomial Witten’s path-integral formulation] for Jones polynomial using Chern-Simons action
*II.  [[Feynmann Diagram]] illustrating [[Associativity]] equation in [[Quantum Field Theory]]  
*II.  [[Feynman Diagram]] illustrating associativity equation in [[Quantum Field Theory]]  
*III. [[Yang-Baxter equation]]
*III. [[Yang-Baxter equation]]
*IV.  [[Lorenz Attractor]]: Lorenz equations with orbit
*IV.  [[Lorenz Attractor]]: Lorenz equations with orbit
*V.  Diagram of a black hole with [[Schwarzschild radius]]
*V.  Diagram of a black hole with [[Schwarzschild radius]]
*VI.  The five [[regular polyhedra]]
*VI.  The five regular polyhedra
*VII. Equiangular spiral drawn in "golden" rectangle (side ratio = golden mean g), ratio of consecutive [[Fibonacci numbers]] approaches g, represented by its continued fraction expansion.
*VII. Equiangular spiral drawn in "golden" rectangle (side ratio = golden mean g), ratio of consecutive [[Fibonacci numbers]] approaches g, represented by its continued fraction expansion.
*VIII.[[Babylonian computation of the square root of 2]]
*VIII.[[Babylonian computation of the square root of 2]]
Line 14: Line 19:
*X.  [[Cell decomposition of torus; Euler characteristic; Gauss-Bonnet formula.]]
*X.  [[Cell decomposition of torus; Euler characteristic; Gauss-Bonnet formula.]]
*XI.  Archimedes: [[On the Sphere and Cylinder]].
*XI.  Archimedes: [[On the Sphere and Cylinder]].
*XII. [[Aharanov-Bohm effect]]
*XII. [[Aharanov-Bohm Effect]]
*XIII.[[Supergravity Langangian]]; root diagramm for [[Lie group E8]]
*XIII.[https://en.wikipedia.org/wiki/Supergravity Supergravity] Langangian; root diagram for [[Lie group E8|Lie Group E8]]
*XIV. [[Navier-Stokes equation]] with flow around cylinder.
*XIV. [[Navier-Stokes equation]] with flow around cylinder.


Line 38: Line 43:
*E. [[Heisenberg's indeterminacy relation]]
*E. [[Heisenberg's indeterminacy relation]]
*F. [[Euler's formula for Zeta-function]]
*F. [[Euler's formula for Zeta-function]]
*G. Interaction between two string; [[Feynman diagram]] shows corresponding interaction of particles, here the Compton scattering of a photon off an electron.
*G. Interaction between two string; [[Feynman Diagram]] shows corresponding interaction of particles, here the Compton scattering of a photon off an electron.


== Questions by Eric Weinstein ==
=== What is $$F_A$$ geometrically? ===
$$F_A$$ is the curvature tensor associated to the connection or vector potential $$A$$.
=== What are $$R_{\mu v}$$ and $$R$$ geometrically? ===
[https://en.wikipedia.org/wiki/Einstein_field_equations Einstein field equations]
Einstein’s original publication, Die Feldgleichungen der Gravitation, in English
==== $$R$$ ====
[https://www.youtube.com/watch?v=UfThVvBWZxM&t=12m6s Explanation of $$R$$]
$$R$$ is a scalar value, describing the "curvature of the spacetime manifold" at each point along the manifold. It's based on a concept of 'parallel transport', where you move a vector around some path on the manifold.
$$R$$ can be computed at each point on the manifold, and describes the difference in the vector's angle after following an infinitesimally small path around the neighborhood of that point, vs. what it was originally. The video does a great job of visualizing when and why that vector angle change would happen, with flat vs. curved manifolds.
In the video, they focus first on the curvature of space. Hopefully they incorporate back in curvature in time, because that's less obvious.
==== $$R_{\mu v}$$ ====
The same video then proceeds to explain $$R_{\mu v}$$. It progresses through some concepts.
===== Computing length in non-orthogonal bases =====
First, just describing the length of a vector on a curved space is hard. It is given by:
$$Length^{squared} = g_{11}dX^{1}dX^{1} + g_{12}dX^{1}dX^{2} + g_{21}dX^{2}dX^{1} + g_{22}dX^{2}dX^{2}$$
Some notes:
* This is not Pythagorean theorem, because $$dX^{1}$$ and $$dX^{2}$$ are not perpendicular.
* Instead, looks like a formula to get the diagonal from two opposite vertices in a parallelogram.
* If $$dX^{1}$$ and $$dX^{2}$$ are perpendicular, then $$g_{12}$$ and $$g_{21}$$ would be 0, and we would get $$Length^{squared} = g_{11}(dX^{1})^{2} + g_{22}(dX^{2})^{2}$$
* See: [https://www.youtube.com/watch?v=UfThVvBWZxM&t=14m27s the video @ 14m27s]
===== Computing vector rotation due to parallel transport =====
Then, they show parallel transport when following a parallelogram, but over a curved 3D manifold. To compute the vector rotation by components, they show:
$$dV^{1} = dX^{1}dX^{2} (V^{1}R^{1}_{112} + V^{2}R^{1}_{212} + V^{3}R^{1}_{312})$$
$$dV^{2} = dX^{1}dX^{2} (V^{1}R^{2}_{112} + V^{2}R^{2}_{212} + V^{3}R^{2}_{312})$$
$$dV^{3} = dX^{1}dX^{2} (V^{1}R^{3}_{112} + V^{2}R^{3}_{212} + V^{3}R^{3}_{312})$$
or, using $$i$$ to summarize across all 3 components (difference vectors):
$$dV^{i} = dX^{1}dX^{2} (V^{1}R^{i}_{112} + V^{2}R^{i}_{212} + V^{3}R^{i}_{312})$$
or , using $$j$$ to index over all 3 components (original vector):
$$dV^{i} = dX^{1}dX^{2} \Sigma_{j} [(V^{j}R^{i}_{j12}]$$
See: [https://www.youtube.com/watch?v=UfThVvBWZxM&t=19m33s the video @ 19m33s]
Open questions:
* Why a parallelogram?
* How to properly overlay the parallelogram onto the 3d manifold, in order to do the parallel transport?
* How does this relate to the length computation above?
===== Putting it all together =====
Now, moving to 4D, we can compute $$R_{\mu v}$$ as:
$$R_{00} = R^{0}_{000} + R^{1}_{010} + R^{2}_{020} + R^{3}_{030}$$
$$R_{10} = R^{0}_{100} + R^{1}_{110} + R^{2}_{120} + R^{3}_{130}$$
$$R_{01} = R^{0}_{001} + R^{1}_{011} + R^{2}_{021} + R^{3}_{030}$$
etc.
Indexing i over all 4 component vectors / dimensions, we get:
$$R_{00} = \Sigma_{i} R^{i}_{0i0}$$
$$R_{10} = \Sigma_{i} R^{i}_{1i0}$$
$$R_{01} = \Sigma_{i} R^{i}_{0i1}$$
etc.
Summarizing on $$\mu$$, we get:
$$R_{\mu 0} = \Sigma_{i} R^{i}_{\mu i0}$$
$$R_{\mu 1} = \Sigma_{i} R^{i}_{\mu i1}$$
etc
Summarizing on $$v$$, we get:
$$R_{\mu v} = \Sigma_{i} R^{i}_{\mu iv}$$
Open questions:
* If we hadn't moved from 3D to 4D, what would this all have looked like?
* What does this have to do with the parallelogram?
* Why are there two indices?
=== How do they relate? ===
[https://en.wikipedia.org/wiki/Cohomology Cohomology]
=== What does this have to do with Penrose Stairs? ===
* [https://en.wikipedia.org/wiki/Penrose_stairs Penrose stairs]
* [https://en.wikipedia.org/wiki/Spinor Spinor]
We’ve heard Eric talk about Penrose stairs and spinors - essentially phenomena where you cannot return to the original state through a 360 degree rotation, but require a 720 degree rotation.
=== What are “Horizontal Subspaces” and what do they have to do with Vector Potentials or Gauge fields? ===
* [https://en.wikipedia.org/wiki/Vertical_and_horizontal_bundles Vertical and horizontal bundles]
* [https://en.wikipedia.org/wiki/Introduction_to_gauge_theory Introduction to gauge theory]
* [https://en.wikipedia.org/wiki/Symmetry_(physics) Symmetry]
From '''theplebistocrat''':
<blockquote>Generally, we're wanting to understand how fermions arise from - or are embedded within / upon - topological "spaces" that have distinct rules which govern operations within those topological spaces, and then how those rules produce higher dimensional operations in corresponding spaces.
Just intuitively, and geometrically speaking, the image that I'm getting when describing all of this and trying to hold it in my head is the image of a sort of Penrose Tower of Babel, where the fundamental underlying structures reach upwards (but also downwards and inwards?) before reaching a critical rotation that corresponds to a collapse of structure into a higher dimensional fiber bundle.
But doesn't this require the symmetry break? How is left and right rotation in a subspace transformed into verticality? This is a crazy rabbit hole, friends. Keep your chins up. Let me know if this was helpful or leading astray.
</blockquote>


== Further Resources ==
== Further Resources ==
Line 175: Line 50:
* [https://www.youtube.com/playlist?list=PL5TiDYF_g45CyK7w7ZXH24FiuASYes2VO Youtube playlist with helpful videos]
* [https://www.youtube.com/playlist?list=PL5TiDYF_g45CyK7w7ZXH24FiuASYes2VO Youtube playlist with helpful videos]
* [http://scgp.stonybrook.edu/archives/6264 List of elements on the Wall at Stony Brook]
* [http://scgp.stonybrook.edu/archives/6264 List of elements on the Wall at Stony Brook]
[[Category:Graph, Wall, Tome]]
[[Category:Projects]]
[[Category:Archive]]

Latest revision as of 00:27, 20 October 2022

  • The Wall

    The Wall

  • Explanations for the Wall

    Explanations for the Wall

The following list contains the names of all equations, formulas, and illustrations that are shown on the Wall. The goal is to create a helpful explanation for each element of the list.




Further Resources[edit]

Retrieved from "https://theportal.wiki/index.php?title=Annotating_the_Wall&oldid=12674"