Pythagorean Theorem

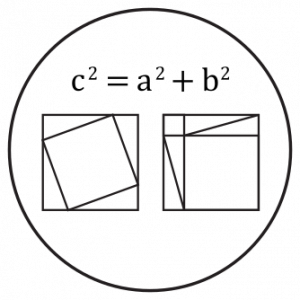
In mathematics, the Pythagorean theorem, also known as Pythagoras' theorem, is a fundamental relation in Euclidean geometry among the three sides of a right triangle. It states that the area of the square whose side is the hypotenuse (the side opposite the right angle) is equal to the sum of the areas of the squares on the other two sides. This theorem can be written as an equation relating the lengths of the sides a, b and c, often called the "Pythagorean equation":

[math]\displaystyle{ a^{2}+b^{2}=c^{2} }[/math]

where [math]\displaystyle{ c }[/math] represents the length of the hypotenuse and [math]\displaystyle{ a }[/math] and [math]\displaystyle{ b }[/math] the lengths of the triangle's other two sides. The theorem, whose history is the subject of much debate, is named for the ancient Greek thinker Pythagoras.

Resources:

• Pythagorean theorem

Discussion:

Anon: I spent a great deal of my career as a student at St. John's College SF thinking about this theorem in particular. To those out there who see this, I offer another portal. If you feel as though you lack the tools to begin your journey, if what you seek is somewhere to begin, I recommend that you attempt to digest the entire first book of Euclid's Elements. I suggest you take it seriously and discuss it with friends, but more importantly, I suggest you display the proofs on a chalkboard, without the book or notes, to anyone you can. When you can teach it to someone else you will have made it further than nearly anyone else, and you might be close to really understanding it. Euclid built one of the first "universal" geometrical models, and he did it with just a few definitions, some common notions, a straight-edge and a compass. It also took us about 1700 years to realize that we could build a complete model with even fewer givens, specifically postulate 5, allowing us our first glimpse of hyperbolic and elliptical space. enjoy!

https://mathcs.clarku.edu/~djoyce/java/elements/bookI/propI47.html